A-Level 시험 숙제 . 23.1 단원

Question 1a:

This question is about the formation of calcium oxide.

Explain the meaning of the term lattice energy.

Question 1b:

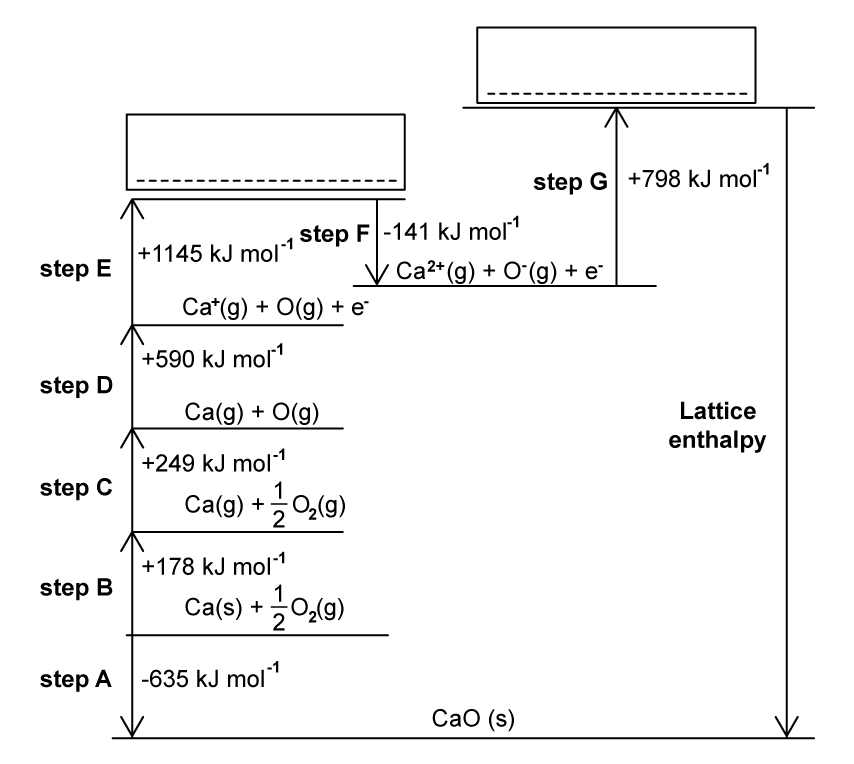
The Born-Haber cycle in Fig. 1.1 can be used to determine the lattice enthalpy of calcium oxide. Steps A-G include the values for enthalpy changes. Complete the Born-Haber cycle by adding the species present on the two dotted lines. Include state symbols.

Question 1c:

Name the enthalpy changes for the following steps in the Born-Haber cycle.

Step B: .....................................................................
Step D: ...................................................................
Step F: ...................................................................

Question 1d:

Step C represents the enthalpy change of atomisation of oxygen. Why is the enthalpy change of atomisation always a positive value?

Question 2a:

This question is about lattice enthalpy.

Write one equation to represent the following changes:

Atomisation of sodium
Second ionisation energy of magnesium
First electron affinity of chlorine

Question 2b:

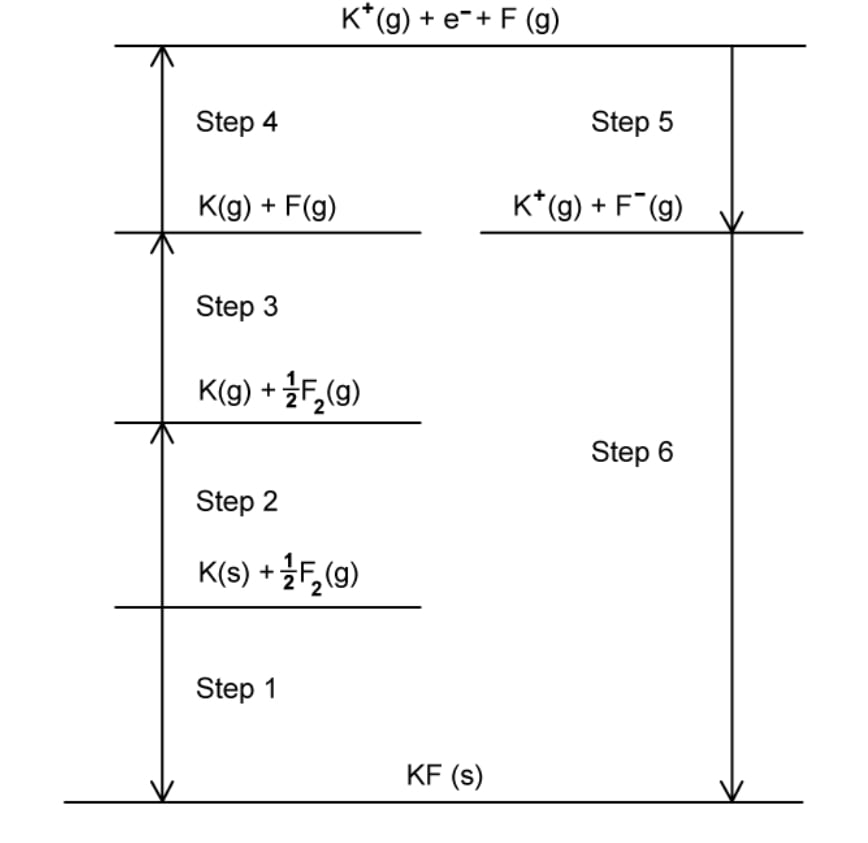
The Born-Haber cycle for the formation of potassium fluoride is shown in Fig. 2.1.

Complete Table 2.1 by naming the enthalpy changes associated with the identified steps.

Question 2c:

The enthalpies of lattice formation for potassium fluoride and caesium fluoride are –830 kJ mol^-1 and -730 kJ mol^-1 respectively.

Explain why the enthalpy of lattice formation is more exothermic for potassium fluoride.

Question 2d:

Use the data in Table 2.2 to calculate the enthalpy of solution of potassium fluoride.

Table 2.2

ΔH_θlatt KF: -830 kJ mol^-1
ΔH_θhyd K⁺: -351 kJ mol^-1
ΔH_θhyd F^–: -504 kJ mol^-1

Question 3a:

This question is about enthalpy changes and calculations.

Table 3.1 shows the enthalpy data for the formation of sodium chloride.

Enthalpy Change	Reaction	Enthalpy Change / kJ mol^-1
ΔH_θf NaCl	Na (s) + ½Cl₂ (g) → NaCl (s)	-411
ΔH_θat Cl	½Cl₂ (g) → Cl (g)	121
ΔH_θat Na	Na (s) → Na (g)	108
E_ea Cl	Cl (g) → Cl^- (g)	-349
ΔH_θie Na	Na (g) → Na⁺ (g)	496
ΔH_θlatt NaCl	Na⁺ (g) + Cl^- (g) → NaCl (s)	To be calculated

Question 3b:

State the definition of electron affinity.

Question 3c:

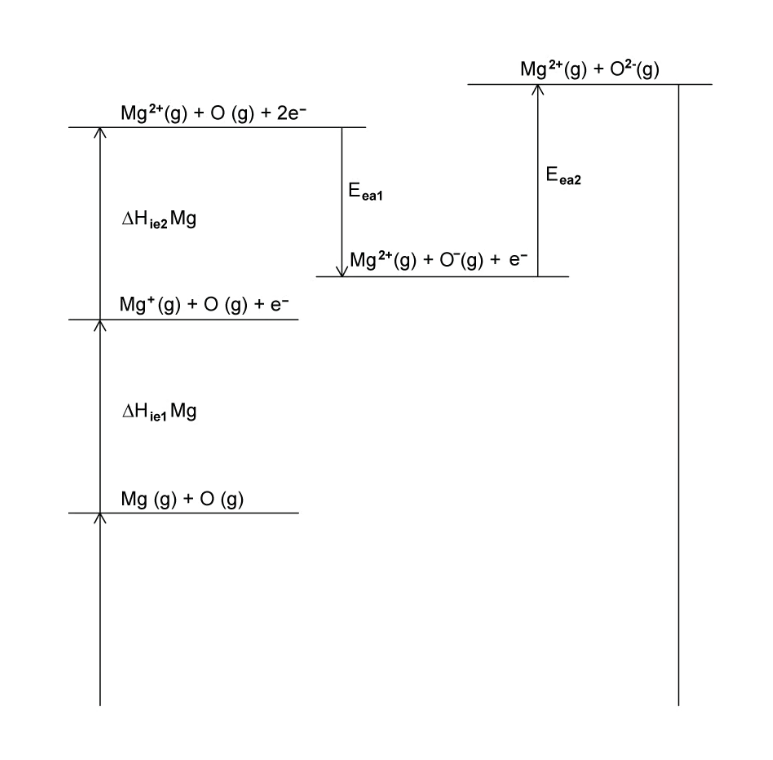
A section of the Born-Haber cycle for the formation of magnesium oxide is shown in Fig. 3.1.

Question 1a:

Lattice energies are always negative showing that they represent exothermic changes.

i) Explain what is meant by lattice energy.

ii) Explain why lattice energy is an exothermic process.

Energy Change	Value / kJ mol^-1
Standard enthalpy change of atomisation of potassium	+89
Electron affinity of O(g)	-141
Electron affinity of O^-(g)	+798
Standard enthalpy change of formation of potassium oxide	-361
First ionisation energy of potassium	+418
Second ionisation energy of potassium	+3070
First ionisation energy of oxygen	+1310
Second ionisation energy of oxygen	+3390
O=O bond energy (diatomic molecule)	+496
O–O bond energy (polyatomic molecule)	+150

Question 1b:

i) Use relevant data from Table 5.1 to calculate the lattice energy of potassium oxide, K₂O (s). Show your working.

ii) State how Na₂O (s) differs from K₂O (s). Indicate this by placing one tick (✔) in the appropriate box in Table 5.2.

Comparison	Na₂O (s) is less negative than K₂O (s)	Na₂O (s) is the same as K₂O (s)	Na₂O (s) is more negative than K₂O (s)
✔

Question 2a:

One use of pure crystals of lithium fluoride is in X-ray monochromators.

The Born-Haber cycle for lithium chloride is shown in Fig. 2.1.

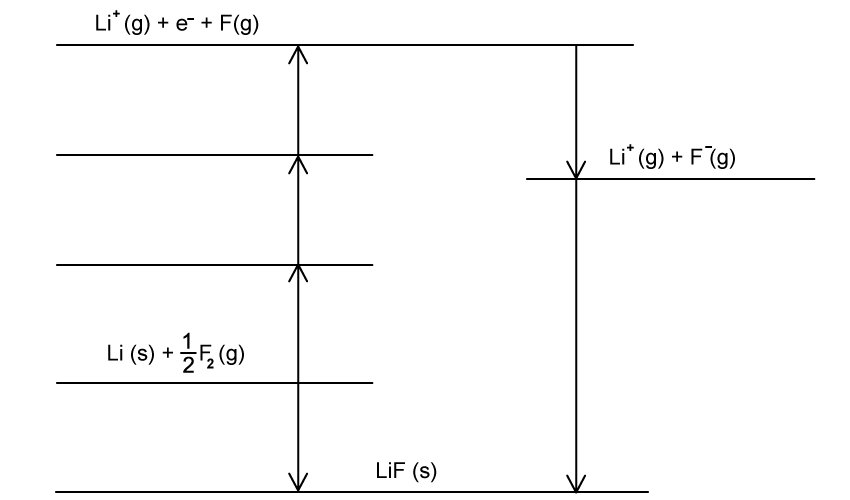
i) Define the term enthalpy of atomisation.

ii) Explain why the enthalpy of atomisation of fluorine is positive.

iii) Complete the Born-Haber cycle for lithium fluoride in Fig 2.1 by adding the missing species on the lines.

Question 2b:

Use the data in Table 2.1 and your completed Born–Haber cycle from part (a) to answer the questions below.

Name of Enthalpy Change	Energy Change / kJ mol^-1
Li (s) → Li (g)	+216
Li (g) → Li⁺ (g) + e^-	+520
F₂ (g) → 2F (g)	+158
F (g) + e^- → F^- (g)	-348
Li (s) + ½F₂ (g) → LiF (s)	-594

Question 2c:

This question is about enthalpy changes in solution.

i) Write the equation for the process showing the enthalpy of solution of potassium fluoride. Include state symbols in your answer.

ii) Use the data in Table 2.2 to calculate the standard enthalpy of solution of potassium fluoride.

Name of Enthalpy Change in Solution	Enthalpy Change (kJ mol^-1)
Enthalpy of lattice dissociation of potassium fluoride	+829
Enthalpy of hydration of potassium ions	-340
Enthalpy of hydration of fluoride ions	-504

Question 2d:

Explain why the value for the enthalpy of hydration, ΔH_θhyd, of Group 1 ions increases from lithium to caesium.

Question 3a:

Calcium chloride, CaCl₂, is an important industrial chemical used in refrigeration plants, for de-icing roads, and for giving greater strength to concrete.

Use an equation to show what is meant by the lattice energy of calcium chloride.

Question 3b:

Explain how the lattice energies of the following salts compare in magnitude with that of calcium chloride:

Calcium fluoride
Calcium sulfide

Question 3c:

Use the data in Table 3.1 to calculate the lattice energy of CaCl₂.

Standard Enthalpy Change	Value / kJ mol^-1
Standard enthalpy change of formation	-796
Standard enthalpy change of atomisation of Ca(s)	+178
Electron affinity per mole of chlorine atoms	-349
First ionisation energy of Ca	+590
Second ionisation energy of Ca	+1150
Standard enthalpy change of atomisation Cl₂ (g)	+244

Question 3d:

When a solution of CaCl₂ is added to a solution of the dicarboxylic acid, malonic acid, the salt calcium malonate is precipitated as a white solid. The solid has the following composition by mass:

Ca: 28.2%
C: 25.2%
H: 1.4%
O: 45.2%

i) Calculate the empirical formula of calcium malonate.

ii) Suggest the structural formula of malonic acid.

Question 4a:

Magnesium reacts with fluorine to form magnesium fluoride.

Explain what is meant by the term first electron affinity.

Question 4b:

i) Write an equation for the second electron affinity of fluorine.

ii) Suggest why the second electron affinity for fluorine is endothermic.

Question 4c:

Determine the electron affinity of a fluorine atom, ΔH_θEA, using Table 5.1.

Name of Enthalpy Change	Energy Change (kJ mol^-1)
Enthalpy of atomisation of magnesium	+150
Second ionisation energy of magnesium	+1450
Enthalpy of formation of magnesium fluoride	-642
Lattice enthalpy of formation of magnesium fluoride	-2493

Question 4d:

Explain the trend in the first electron affinities going from fluorine to iodine.

Question 1a:

Potassium sulfide is a reagent used in analytical chemistry and pharmaceutical preparations. Draw a fully labelled Born-Haber cycle for the formation of solid potassium from its elements. Include state symbols for all species involved.

Question 1b:

Use the data in Table 1.1 to calculate the lattice enthalpy of potassium sulfide, ΔH_θlatt. Show your working.

Enthalpy Change	Enthalpy Change / kJ mol^-1
Formation of potassium sulfide	-381
1st electron affinity of sulfur	-200
2nd electron affinity of sulfur	+640
Atomisation of sulfur	+279
1st ionisation energy of potassium	+419
Atomisation of potassium	+89

Question 1c:

Explain the difference in values between the first and second electron affinities of sulfur.

Question 2a:

Magnesium oxide is often used in optical applications due to its light-reflecting properties in crystal form.

Write an equation to represent the lattice energy of MgO.

Question 2b:

Use the data in Table 2.1 to calculate a value for the second ionisation energy of magnesium, ΔH_θie. Show your working.

Enthalpy Change	Enthalpy Change / kJ mol^-1
Lattice energy of MgO (s)	-3791
Enthalpy change of atomisation of Mg	+148
Enthalpy of atomisation of oxygen	+248
Electron affinity of the oxygen atom	-141
Electron affinity of the oxygen anion, O^-	+798
First ionisation energy of Mg	+736
Enthalpy of formation of MgO	+552

Question 2c:

Magnesium oxide is generally insoluble in water whereas calcium oxide is sparingly soluble. Explain why there is no enthalpy of solution data for calcium oxide.

Question 3a:

The incomplete Born-Haber cycle for sodium selenide is shown below in Fig. 3.1.

Write the equations for processes 1, 2 and 3.

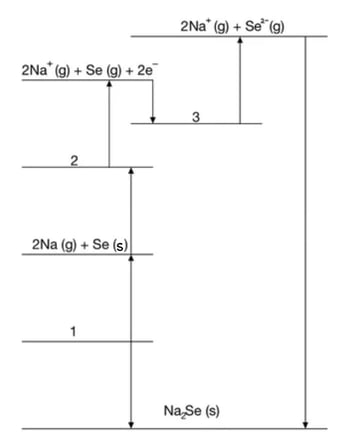
Question 3b:

If sulfur is used as opposed to selenium in the lattice, suggest the change in value of the lattice energy, ΔH_θlatt. Explain your answer.

Question 3c:

Use the data in Table 3.1 to calculate the lattice energy of aluminium oxide, ΔH_θlatt. Show your working.

Enthalpy Change	Enthalpy Change / kJ mol^-1
Atomisation of aluminium	+326
Atomisation of oxygen	+249
First ionisation energy of aluminium	+578
Second ionisation energy of aluminium	+1817
Third ionisation energy of aluminium	+2745
Electron affinity of O atom	-141
Electron affinity of O^-	+753
Formation of aluminium oxide	-1670

A-Level 시험 숙제 . 23.1 단원

Question 1a:

Question 1b:

Question 1c:

Question 1d:

Question 2a:

Question 2b:

Question 2c:

Question 2d:

Question 3a:

Question 3b:

Question 3c:

Question 1a:

Question 1b:

Question 2a:

Question 2b:

Question 2c:

Question 2d:

Question 3a:

Question 3b:

Question 3c:

Question 3d:

Question 4a:

Question 4b:

Question 4c:

Question 4d:

Question 1a:

Question 1b:

Question 1c:

Question 2a:

Question 2b:

Question 2c:

Question 3a:

Question 3b:

Question 3c:

댓글 쓰기